The Giza Pyramid and Root Numbers -
A Different Perspective
Disclaimer: My approach focuses on aesthetic inspiration, practicality, and real-life theoretical implications rather than mathematical rigor.
Did the Egyptians possess knowledge of the division of surfaces according to the golden ratio?
I recently revisited some old notes from 1995 in which I analyzed the Giza Pyramid using a root rectangle perspective. I quickly found that the angles and proportions were closely related to the "extreme mean ratio" in every angle and corner. At that time, I couldn't believe how well the measurements aligned, so I put the notes aside. Upon revisiting these notes, I found that the numbers indeed aligned quite well.
In this article, I will attempt to demonstrate these relationships in a way that suggests the Egyptians may have known how to calculate the division of surfaces according to the golden ratio, or more accurately, root numbers. I propose that their knowledge extended beyond the simple 3:4:5 triangle to a deeper understanding of geometry, and possibly even trigonometry. Furthermore, it appears that they built the pyramid using a strict formula centered around the golden ratio. I'm not suggesting they used calculators as I do; all that is needed for my demonstration is sticks and strings, as that is how root rectangles can be constructed (read more about this further down or in my article "A Closer Look at Root Rectangles").
First, let's examine the well-known 3:4:5 triangle and why I believe it was not the Egyptians' only geometric tool.
 |
 |
| 3:4:5 Triangle | 1.618 Triangle |
The 3:4:5 triangle is an excellent tool for creating 90° angles. It can be easily confused with the flipped cross-section of the pyramid due to their similarities. I have converted the numbers to a root rectangle perspective to clarify the comparison.
In Figure 2, we see a similar but distinctly different triangle. The dimensions of the square shapes are no longer nice, even regular numbers. However, what caught my attention is that it matches the dimensions of the cross-section of the pyramid much better than the 3:4:5 triangle.
1 * 220 = 220 (1/2 the base)
1.272 * 220 ≈ 280 (pyramid height)
1.618 * 220 ≈ 356 (hypotenuse)
1.667 ≠ 1.618 (it would make a difference of several meters on the Giza pyramid)
Others have already discovered what I found, and it has been debated and disputed whether the Egyptians used the 1:0.618 / 1:1.618 formula deliberately or by chance. The purpose of this article is not to prove anything right or wrong but to present an experiment to see if constructing the pyramid this way is plausible.
I want to emphasize that I am not claiming that the Egyptians used these numbers for any divine, alien intelligence, or cosmic knowledge reason. I don't know why the numbers or angles line up as well as they do, but I will try to demonstrate this from a root rectangle perspective.
I am not a mathematician, and I don't think one needs to be to calculate the angles and relationships I'm illustrating. Root numbers provide the simplest way to divide surfaces into components and relationships that are scalable, with each surface relating in proportion, area, and line divisions to the other elements in the same 3-dimensional shape.
Let's start with the measurements. This is what I have found in different literature and on the web.
The Numbers
Measurements
| Height | 280 (146.64) , now approximately 262 (137.2) |
| Lengths of sides | North 439.67(230.25) , south 440.05 (230.25)
East 439.93 (230.39) , west 439.87 (230.36) Now approximately 434 (227.29) |
| Angles of corners | North east 90° 3' 2" , north west 89° 59' 58"
South east 89° 56' 27" , south west 90° 0' 33" |
| Orientation | Average deviation of sides from cardinal directions 3' 6"
Base is level to within 0.04 (0.021) |
Measurements Approx
| cubits | Believed | Current | |
| Base Width | 440 | 230.37 metres | ? |
| Height | 280 | 146.478 metres | 138.75 metres |
http://en.wikipedia.org/wiki/Great_Pyramid_of_Giza
With these measurements in mind, the hypotenuse of the cross-section relates to the base width/2 with a ratio of 0.618.
If we simplify the number and set the base width of the pyramid to 2 units some interesting numbers comes up. Anyone familiar with the "golden cut" recognizes the number 0.168. Have a look at this:
sin α = (base / 2) / hypotenuse = 220 / 356.09 ≈ 0.618
Let's check this using the Unit circle
In this example the cross-section is flipped on the side for the sole purpose of readability when inscribed in a "Unit circle". The unit refers to the hypotenuse and this is always 1. With numbers we know this will be:
356.09 / 356.09 = 1 unit
tan α = y / x = 220 / 280 ≈ 0.786
y = sin α = y / 1 ≈ 0.618
x = cos α = x / 1 ≈ 0.786
The angle of t in degrees (not really interesting in this scenario)
t = atan( y / x) * (180/pi) ≈ 38.2°
With this we can see that the hypotenuse multiplied with y (the base) will be 1 * 0.618 = 0.618 (not so strange). But, consider this (using the number we know):
356.09 * 0.618 ≈ 220
What I'm trying to show is this number 0.618, so please bare with me. I will try to explain this in greater detail further on.
Have a look at this:
220 * 1.618 ≈ 356
which means
base * 1.618 = hypotenuse
If we want the base to be the unit number (1) the hypotenuse will be 1.618, like this:
1 * 1.618 = 1.618
(The unit 1 represents 220 cubits.)
Explanation of the formula using Pythagoras theorem:
hypotenuse = √1(unit)2 + height2
or with the real cubits values (down-sampled so that 220 represent the value of 1)
1.618 ≈ √(220/220)2 + (280.0/220)2
Why these decimal numbers? It is irrational! The Egyptians did not have calculators the same we do. The reason I am using numbers is to see if the geometry checks out. The thing is, a calculator is not a very good tool for this. A better way is to use pen, paper, needles and a piece of string. As stated earlier, all this can be done with sticks and strings. Let me try to tell you how.
0.618 The Stick And String Way
Take a look at this image

Forget about calculations for a while. Let us say we make a straight line using a string between two marks. Let this be the unit 1 (it can be any length) and this will be the starting point for for the rest of the layout. Then create a right angled square. Use the diagonal to draw an arch so that it hits the base line. The newly formed rectangle has a ratio of 1:√2. By repeating the same procedure we have a new rectangle with the ratio of 1:√3 and so on. Think about this: You don't have to know anything about Pythagoras or any other theorem to be able to do this. It is just a very practical way of working with surface layouts and surface divisions. I am showing this with numbers and symbols but you can put those away as well. It will still work with using just sticks and strings. We are working with areas (surfaces) rather than numbers here. This is how root rectangles can be understood in the most simplistic way. It is dealing with surfaces (areas) rather than straight lines. And this is also one of the reasons why the proportions usually don't come up nice and even as integers. Root numbers often produce irrational numbers.
If you want to learn more about this, read the "A Closer Look at Root Rectangles" article and have a look at this interactive proof of the Pythagorean theorem.
Let us continue with the same rectangle for a while and see what happens.
We have used the diagonal from the √3 rectangle, created a √4 rectangle (same as 1+1) and have finally created a √5 rectangle.
If you understand what root rectangles are by now, take a look at this:
φ = ( √5 - 1 ) / 2 ≈ 0.618
or
φ = ( 1 + √5.0 ) / 2 ≈ 1.618
Here are these numbers again!
The interesting thing about this irrational number 0.618 and 1.618 is that the unit 1 relates to 0.618 as 1.618 to 1. In ancient Greece this ratio was called "phi" or "φ". This ratio was also know as dividing a line in the extreme and mean ratio. In more general terms this ratio is also known as the "golden mean", "golden ratio", "golden section", golden cut", "golden number, "divine proportion", … Read more about it here under the section "A closer look at 1:√5".

A Closer Look At The Sections
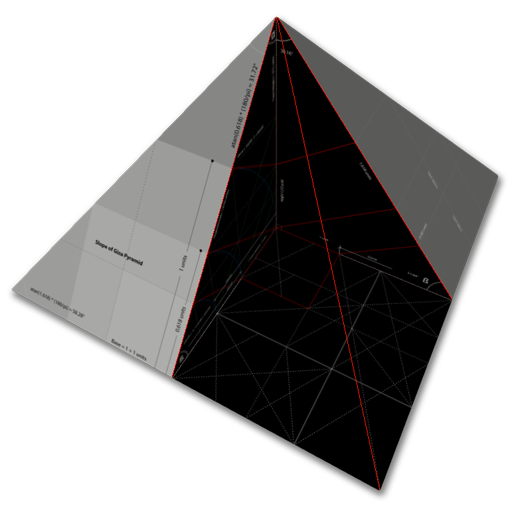
Let us unfold the pyramid. What you see in the image below is a cross cut of the Giza pyramid. If you have a decent browser, meaning updated versions of Firefox, Chrome or Safari (older versions of Internet Explorer will not work), you can check this interactive 3D model in WebGL, visually describing my theories about the Giza Pyramids layout.
W.I.P.
Height Projection
The black triangle is the cross-section of the pyramid and the hypotenuse in the blue square is the slope.
The 1 unit represents 220 cubits.
Please note that this is the slope, not the cross-section. The 1 unit represents 220 cubits.
© Hans E Andersson
Related documents
A Closer Look at Root Rectangles